
arXiv:math-ph/0702087v4 30 May 2021
Application of Analytic Functions to the Global
Solvabilty of the Cauchy Problem for Equations of
Viscous Incompressible Liquid
Durmagambetov A.A
1
a
010000, Kazakhstan
Abstract
Using the example of a complicated problem such as the Cauchy problem for the
Navier–Stokes equation, we show how the Poincar´e–Riemann–Hilbert boundary-
value problem enables us to cons truct effective estimates of solutions for this
case. The apparatus of the three-dimensional inverse problem of quantum scat-
tering theory is develop ed for this.
Keywords: Riemann, Hilbert, Poincar´e, Cauchy, the Navier–Stokes equa tions
2010 MSC: 76D05
1. Intro duction
Using the example of a complicated problem such as the Cauchy problem
for the Navier–Stokes equation, we s how how the Poincar´e–Riemann–Hilbert
boundary-value problem enables us to construct effective estimates of solutio ns
for this case. The apparatus of the three -dimensional inverse problem of q uan-
tum scattering theory is develop e d for this. It is shown that the unitary scat-
tering operator ca n be studied as a solution of the Poincar´e–Riemann–Hilbert
boundary-value problem. This allows us to go on to s tudy the potential in the
Schr¨odinger equation, which we consider as a velocity c omp onent in the Navier–
Stokes equatio n. The same scheme of reduction of Riemann integral equations
Email address: aset.durmagambet@gmail.com (Durmagambetov A.A)
URL: (Durmagambetov A.A)
1
Preprint submitted to Journal of L
A
T
E
X Templates June 1, 2021
for the zeta function to the Poincar´e–Riemann–Hilbert boundary-value prob-
lem allows us to constr uc t effective estimates that describe the behaviour of the
zeros of the zeta function very well.
2. Results for the one-dimensional case
Let us consider a one-dimensional function f a nd its Fourier transformation
˜
f. Using the notions of module and phase, we write the Fourier transfo rmation
in the following form:
˜
f = |
˜
f|exp(iΨ) , where Ψ is the phase. The Plancherel
equality states that ||f||
L
2
= const||
˜
f||
L
2
. Here we can see that the phase does
not contribute to determination of the X norm. To estimate the ma ximum we
make a simple estimate as max|f|
2
≤ 2||f||
L
2
||∇f||
L
2
. Now we have an estimate
of the function maximum in which the phase is not involved. Let us consider
the behaviour of a progressing wave travelling with a constant velocity of v = a
described by the function F (x, t) = f (x + at). Its Fourier transformation with
respect to the variable x is
˜
F =
˜
fexp(iatk). Again, in this case, we can see
that when we study a module of the Fourier transformation, we will not obtain
major physical information about the wave, such as its velocity and location of
the wave crest because |
˜
F | = |
˜
f| . T he se two examples show the weaknesses of
studying the Fourier transformation. Many researchers focus on the study of
functions using the embedding theorem, in which the main object of the study
is the module of the function. Howeve r, as we have seen in the given examples,
the phase is a principal physical characteristic of any process, and as we can see
in mathematical studies that use the embedding theorem with energy estimates,
the phase disappears. Along with the phase, all reasonable information about
the physical process disappears, as demo nstrated by Tao [1] and other research
studies. In fac t, Tao built progre ssing waves that are not followed by energy
estimates . Let us proceed with a more essential analy sis of the influence of the
phase on the behaviour of functions.
Theorem 1. There are functions of W
1
2
(R) with a constant rate of the norm for
a gradient catastrophe for which a phase change of its Fourier transformation
2

is sufficient.
Proof: To prove this, we consider a sequence of testing functions
˜
f
n
=
∆/(1 + k
2
), ∆ = (i − k)
n
/(i + k)
n
. It is obvious that |
˜
f
n
| = 1/(1 + k
2
) and
max|f
n
|
2
≤ 2||f
n
||
L
2
||∇f
n
||
L
2
≤ const. Calculating the Fourier transformation
of these testing functions, we obtain
f
n
(x) = x(−1)
(n−1)
2π exp(−x)L
1
(n−1)
(2x)if x > 0 , f
n
(x) = 0 if x ≤ 0, (1)
where L
1
(n−1)
(2x) is a Laguerre polynomial. Now we see that the functions a re
equibounded and derivatives of these functions will grow with the growth of
n. Thus, we have built an example o f a sequence of the bounded functions of
W
1
2
(R) which have a constant norm W
1
2
(R), and this sequence converges to a
discontinuous function.
The results show the flaws of the embedding theorems when analyzing the be-
havior of functions. Therefore, this work is devoted to overcoming them and
the basis for solving the formulated problem is the analytical properties of the
Fourier transforms of functions on compact sets. Analytical prop e rties and es -
timates of the Fourier transform of functions are studied using the Poincar´e –
Riemann – Hilbert boundary value pro blem
3. Results for the three-dimensional case
Consider Schr¨odinger’s equation:
−∆
x
Ψ + qΨ = k
2
Ψ, k ∈ C. (2)
Let Ψ
+
(k, θ, x) be a solution of (2) with the following asymptotic behaviour:
Ψ
+
(k, θ, x) = Ψ
0
(k, θ, x) +
e
ik|x|
|x|
A(k, θ
′
, θ) + 0
1
|x|
, |x| → ∞, (3)
where A(k, θ
′
, θ) is the scattering amplitude and θ
′
=
x
|x|
, θ ∈ S
2
for k ∈
¯
C
+
=
{Imk ≥ 0} Ψ
0
(k, θ, x) = e
ik(θ,x)
:
A(k, θ
′
, θ) = −
1
4π
Z
R
3
q(x)Ψ
+
(k, θ, x)e
−ikθ
′
x
dx.
3

Solutions to (2) and (3) are obtained by solving the integral equation
Ψ
+
(k, θ, x) = Ψ
0
(k, θ, x) +
Z
R
3
q(y)
e
+ik|x−y|
|x − y|
Ψ+(k, θ, y)dy = G(qΨ
+
),
which is called the Lippman–Schwinger equation.
Let us introduce
θ, θ
′
∈ S
2
, Df = k
Z
S
2
A(k, θ
′
, θ)f (k, θ
′
)dθ
′
.
Let us also define the solution Ψ
−
(k, θ, x) for k ∈
¯
C
−
= {Imk ≤ 0} as
Ψ
−
(k, θ, x) = Ψ
+
(−k, −θ, x).
As is well known [8],
Ψ
+
(k, θ, x) − Ψ
−
(k, θ, x) = −
k
4π
Z
S
2
A(k, θ
′
, θ)Ψ
−
(k, θ
′
, x)dθ
′
, k ∈ R. (4)
This equation is the key to solving the inverse s cattering problem and was first
used by Newton [8,9] and Somersalo e t al. [10].
Definition 1. The set of measurable functions R with the norm defined by
||q||
R
=
s
Z
R
6
q(x)q(y)
|x − y|
2
dxdy < ∞
is recognised as being of Rollnik class.
Equation (4) is equivalent to the following:
Ψ
+
= SΨ
−
,
where S is a scattering o perator with the kernel
S(k, l) =
Z
R
3
Ψ
+
(k, x)Ψ
∗
−
( l, x)dx.
The following theorem was stated in [9]:
Theorem 2. (Energy and momentum conservation laws) Let q ∈ R.
Then, SS
∗
= I and S
∗
S = I, where I is a unitary operator.
4
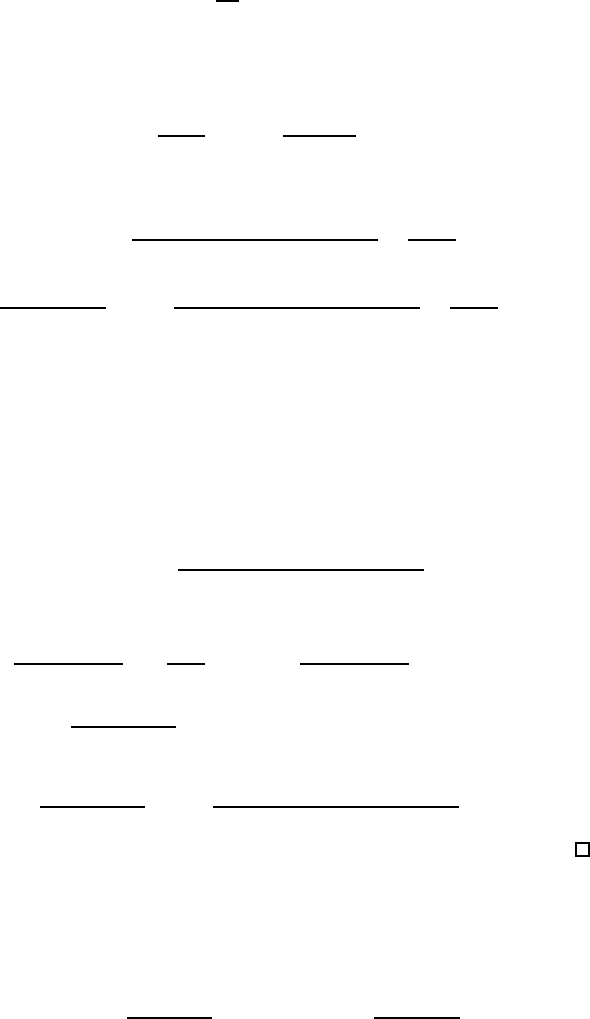
Corollary 1. SS
∗
= I and S
∗
S = I yield
A(k, θ
′
, θ) − A(k, θ, θ
′
)
∗
=
ik
2π
Z
S
2
A(k, θ, θ
′′
)A(k , θ
′
, θ
′′
)
∗
dθ
′′
.
Theorem 3. (Birmann–Schwinger estimation) Let q ∈ R. Then, the
number of discrete eigenvalues can be estimated as
N(q) ≤
1
(4π)
2
Z
R
3
Z
R
3
q(x)q(y)
|x − y|
2
dxdy.
Lemma 1. Let
|q|
L
1
(R
3
)
+ 4π|q|
L
2
(R
3
)
< α < 1/2. Then,
kΨ
+
k
L
∞
≤
|q|
L
1
(R
3
)
+ 4π|q|
L
2
(R
3
)
1 −
|q|
L
1
(R
3
)
+ 4π|q|
L
2
(R
3
)
<
α
1 − α
,
∂(Ψ
+
− Ψ
0
)
∂k
L
∞
≤
|q|
L
1
(R
3
)
+ 4π|q|
L
2
(R
3
)
1 −
|q|
L
1
(R
3
)
+ 4π|q|
L
2
(R
3
)
<
α
1 − α
.
Proof. By the Lippman– Schwinger equation, we have
|Ψ
+
− Ψ
0
| ≤ |GqΨ
+
|,
|Ψ
+
− Ψ
0
|
L
∞
≤ |Ψ
+
− Ψ
0
|
L
∞
|Gq| + |Gq|,
and, finally,
|Ψ
+
− Ψ
0
| ≤
|q|
L
1
(R
3
)
+ 4π|q|
L
2
(R
3
)
1 −
|q|
L
1
(R
3
)
+ 4π|q|
L
2
(R
3
)
.
By the Lippman–Schwinger equation, we also have
∂ (Ψ
+
− Ψ
0
)
∂k
≤
∂Gq
∂k
Ψ
+
+
Gq
∂ (Ψ
+
− Ψ
0
)
∂k
+ |Gq|,
∂(Ψ
+
− Ψ
0
)
∂k
≤
|q|
L
1
(R
3
)
+ 4π|q|
L
2
(R
3
)
,
∂(Ψ
+
− Ψ
0
)
∂k
L
∞
≤
|q|
L
1
(R
3
)
+ 4π|q|
L
2
(R
3
)
1 −
|q|
L
1
(R
3
)
+ 4π|q|
L
2
(R
3
)
,
which completes the proof.
Let us introduce the following notatio n:
Q(k, θ, θ
′
) =
Z
R
3
q(x)e
ik(θ−θ
′
)x
dx, K(s) = s, X(x) = x,
T
+
Q =
Z
+∞
−∞
Q(s, θ, θ
′
)
s − t − i0
ds, T
−
Q =
Z
+∞
−∞
Q(s, θ, θ
′
)
s − t + i0
ds.
5

Lemma 2. Let q ∈ R ∩ L
1
(R
3
), kqk
L
1
+ 4π|q|
L
2
(R
3
)
< α < 1/2. Then,
kA
+
k
L
∞
< α +
α
1 − α
,
∂A
+
∂k
L
∞
< α +
α
1 − α
.
Proof. Multiplying the Lippman–Schwinger equation by q(x)Ψ
0
(k, θ, x) and then
integrating, we have
A(k, θ, θ
′
) = Q(k, θ, θ
′
) +
Z
R
3
q(x)Ψ
0
(k, θ, x)GqΨ
+
dx.
We can estimate this latest equation as
|A| ≤ α + α
|q|
L
1
(R
3
)
+ 4π|q|
L
2
(R
3
)
1 −
|q|
L
1
(R
3
)
+ 4π|q|
L
2
(R
3
)
.
Following a similar procedure for
∂A
+
∂k
completes the proof.
We define the operators T
±
, T for f ∈ W
1
2
(R) as follows:
T
+
f =
1
2πi
lim
Imz→0
∞
Z
−∞
f(s)
s − z
ds, Im z > 0, T
−
f =
1
2πi
lim
Imz→0
∞
Z
−∞
f(s)
s − z
ds, Im z < 0,
T f =
1
2
(T
+
+ T
−
)f.
Consider the Riemann problem of finding a function Φ that is analytic in the
complex plane with a cut along the real axis. Values o f Φ on the two sides of
the c ut a re denoted as Φ
+
and Φ
−
. The following presents the results of [12]:
Lemma 3.
T T =
1
4
I, T T
+
=
1
2
T
+
, T T
−
= −
1
2
T
−
, T
+
= T +
1
2
I, T
−
= T −
1
2
I, T
−
T
−
= −T
−
.
Denote
Φ
+
(k, θ, x) = Ψ
+
(k, θ, x)−Ψ
0
(k, θ, x), Φ
−
(k, θ, x) = Ψ
−
(k, −θ, x)−Ψ
0
(k, θ, x),
g(k, θ, x) = Φ
+
(k, θ, x) − Φ
−
(k, θ, x)/
6

Lemma 4. Let q ∈ R, N(q) < 1, g
+
= g(k, θ, x), and g
−
= g(k, −θ, x). Then,
Φ
+
(k, θ, x) = T
+
g
+
+ e
ikθx
, Φ
−
(k, θ, x) = T
−
g
+
+ e
ikθx
.
Proof. The proof of the above fo llows from the classic results for the Riemann
problem.
Lemma 5. Let q ∈ R, N(q) < 1, g
+
= g(k, θ, x), and g
−
= g(k, −θ, x), ).
Then,
Ψ
+
(k, θ, x) = (T
+
g
+
+ e
ikθx
), Ψ
−
(k, θ, x) = (T
−
g
−
+ e
−ikθx
).
Proof. The pr oof of the above follows from the definitions of g, Φ
±
, and Ψ
±
.
Lemma 6. Let
sup
k
∞
Z
−∞
pA(p, θ
′
, θ)
4π(p − k + i0)
dp
< α,
Z
S
2
αdθ < 1/2.
Then,
Y
0≤j<n
Z
S
2
Z
∞
−∞
k
j
A(k
j
, θ
′
k
j
, θ
k
j
)
4π(k
j+1
− k
j
+ i0)
dk
j
dθ
k
j
≤ 2
−n
.
Proof. Denote
α
j
=
V p
Z
∞
−∞
k
j
A(k
j
, θ
′
k
j
, θ
k
j
)
4π(k
j+1
− k
j
+ i0)
dk
j
,
Therefore,
Y
0≤j<n
Z
S
2
Z
∞
−∞
k
j
A(k
j
, θ
′
k
j
, θ
k
j
)
4π(k
j+1
− k
j
+ i0)
dk
j
dθ
k
j
≤
Y
0≤j<n
Z
S
2
α
j
dθ
k
j
< 2
−n
.
This completes the proof.
Lemma 7. Let
sup
k
Z
S
2
|T
−
QK|dθ ≤ α <
1
2C
< 1, sup
k
Z
S
2
|T
−
˜qK|dθ ≤ α <
1
2C
< 1,
sup
k
Z
S
2
T
−
Q˜qK
2
dθ ≤ α <
1
2C
< 1.
7

Then,
sup
k
Z
S
2
|T
−
AK|dθ ≤
C
R
S
2
|T
−
QK|dθ
1 − sup
k
R
S
2
|T
−
A˜qK
2
|dθ
,
sup
k
Z
S
2
T
−
A˜qK
2
dθ
≤
C
T
−
R
S
2
Q˜qK
2
dθ
1 −
T
−
R
S
2
˜qKdθ
.
Proof. By the definition of the amplitude and Lemma 4, we have
A(k, θ
′
, θ) = −
1
4π
Z
R
3
q(x)Ψ
+
(k, θ, x)e
−ikθ
′
x
dx
= −
1
4π
Z
R
3
q(x)
h
e
ikθ
′
x
+ T
+
g(k, θ, θ
′
)
i
e
−ikθ
′
x
dx.
We can rewrite this as
A(k, θ
′
, θ) = −
1
4π
Z
R
3
q(x)
e
ikθx
+
X
n≥0
(−T
−
D)
n
Ψ
0
e
−ikθ
′
x
dx. (5)
Lemma 6 yields
sup
k
Z
S
2
|T
−
AK|dθ ≤ sup
k
Z
S
2
1
4π
T
−
QK
dθ+
sup
k
R
S
2
|T
−
KA|dθ
2
R
S
2
T
−
A˜qK
2
dθ
1 − sup
k
R
S
2
|T
−
KA|dθ
2
.
Owing to the smallness of the terms on the right-hand side, the following
estimate follows:
sup
k
Z
S
2
|T
−
AK|dθ ≤ 2 sup
k
Z
S
2
1
4π
T
−
QK
dθ.
Similarly,
sup
k
Z
S
2
T
−
A˜qK
2
dθ ≤ C
Z
S
2
T
−
Q˜qK
2
dθ +
Z
S
2
T
−
A˜qK
2
dθ
Z
S
2
|T
−
˜qK|dθ,
sup
k
Z
S
2
T
−
A˜qK
2
dθ ≤
C
R
S
2
T
−
Q˜qK
2
dθ
1 −
R
S
2
|T
−
˜qK|dθ
,
sup
k
Z
S
2
T
−
A˜qK
2
dθ ≤ 2 sup
k
Z
S
2
1
4π
T
−
Q˜qK
2
dθ.
This completes the proof.
8

To simplify the writing of the fo llowing calculations, we introduce the se t
defined by
M
ǫ
(k) =
s|ǫ < |s| + |k − s| <
1
ǫ
.
The Heaviside function is given by
Θ(x) = {1, if x > 0, −1 if x < 0 }.
Lemma 8. Let q, ∇q ∈ ∩L
2
(R
3
), |A| > 0. Then,
πi
Z
R
3
Θ(A)e
ik|x|A
q(x)dx = lim
ǫ→0
Z
s∈M
ǫ
(k)
Z
R
3
e
is|x|A
k − s
q(x)dxds,
πi
Z
R
3
Θ(A)ke
ik|x|A
q(x)dx = lim
ǫ→0
Z
s∈M
ǫ
(k)
Z
R
3
s
e
is|x|A
k − s
q(x)dxds.
Proof. The lemma c an be proved by the conditions of lemma and the le mma of
Jordan.
Lemma 9. Let
I
0
= Ψ
0
(x, k)|
r=r
0
.
Then
Z
+∞
−∞
Z
S
2
Z
S
2
˜q(k(θ − θ
′
))I
0
k
2
dkdθdθ
′
≤ sup
x∈R
3
|q(x)| + C
0
(
1
r
0
+ r
0
) kqk
L
2
(R
3
)
,
sup
θ∈S
2
Z
+∞
−∞
Z
S
2
Z
S
2
QT KQI
0
k
2
dθ
′′
dθ
′
dk
≤ C
0
(
1
r
0
+ r
0
) kqk
2
L
2
(R
3
)
.
Proof. By the definition of the Fourier transform, we have
Z
+∞
−∞
Z
S
2
Z
S
2
˜q(k(θ−θ
′
))I
0
k
2
dkdθdθ
′
=
Z
+∞
−∞
Z
S
2
Z
S
2
Z
+∞
0
q(x)e
ikx(θ−θ
′
)
e
ix
0
k
k
2
dkdθdθ
′
drdγ,
where x = r γ The lemma of Jordan completes the proof for the first inequality.
The second inequality is proved like the first:
V p
Z
+∞
−∞
Z
S
2
Z
S
2
QT KQI
0
k
2
dθ
′′
dθ
′
dk
= V p
Z
+∞
−∞
Z
+∞
−∞
Z
S
2
Z
S
2
Z
S
2
(˜q(s cos(θ
′
) − s cos(θ
′′
))˜q(k cos(θ) − s cos(θ
′′
)) s
k − s
I
0
k
2
dθ
′
dθ
′′
dθdkds.
9

Lemma 8 yields
Z
+∞
−∞
Z
S
2
Z
S
2
Z
S
2
(˜q(k cos(θ
′
) − k cos(θ))˜q(k cos(θ) − k cos(θ
′′
)) I
0
k
3
Θ(cos(θ
′′
))dθ
′
dθ
′′
dθdk−
Z
+∞
−∞
Z
S
2
Z
S
2
Z
S
2
(˜q(k cos(θ
′
) − k cos(θ))˜q(k cos(θ) − k cos(θ
′′
)) I
0
k
3
Θ(−cos(θ
′′
))dθ
′
dθ
′′
dθdk.
Integrating θ, θ
′
, θ
′′
, and k, we obtain the proof of the second inequality of the
lemma.
Lemma 10. Let
sup
k
|T
−
QK| ≤ α <
1
2C
< 1, sup
k
|T
−
˜qK| ≤ α <
1
2C
< 1,
sup
k
T
−
Q˜qK
2
≤ α <
1
2C
< 1, l = 0, 1, 2.
Then,
Z
+∞
−∞
Z
S
2
Z
S
2
A(k, θ
′
, θ)k
l
dkdθ
′
dθ
≤
Z
+∞
−∞
Z
S
2
Z
S
2
˜q(k(θ − θ
′
))k
l
dkdθ
′
dθ
+C sup
θ∈S
2
Z
+∞
−∞
Z
S
2
Z
S
2
QT KAk
l
dθ
′′
dθ
′
dk
,
Z
+∞
−∞
Z
S
2
Z
S
2
A(k, θ
′
, θ)k
2
dkdθ
′
dθ
≤ sup
x∈R
3
|q|+C
0
kqk
W
1
2
(R
3
)
kqk
L
2
(R
3
)
Z
S
2
T KAdθ
′′
+ 1
.
Proof. Using the definition of the amplitude, Lemmas 3 and 4, and the lemma
of Jordan yields
Z
+∞
−∞
Z
S
2
Z
S
2
A(k, θ
′
, θ)k
l
dkdθ
′
dθ = −
Z
+∞
−∞
1
4π
Z
S
2
Z
S
2
Z
R
3
q(x)Ψ
+
(k, θ, x)e
−ikθ
′
x
k
l
dxdkdθ
′
=
−
1
4π
Z
S
2
Z
S
2
Z
R
3
q(x)
e
ikθx
+
X
n≥1
(−T
−
D)
n
Ψ
0
e
−ikθ
′
x
k
l
dθ
′
dxdk
=
Z
+∞
−∞
Z
S
2
Z
S
2
˜q(k(θ − θ
′
))k
l
dkdθ
′
dθ +
X
n≥1
W
n
,
W
1
= V p
Z
R
3
Z
+∞
−∞
Z
S
2
Z
S
2
sA(s, θ
′′
, θ)e
−ikθ
′
x
q(x)e
isθ
′′
x
k − s
k
l
dkdxdsdθ
′
dθ
′′
,
10

|W
1
| ≤ C sup
θ∈S
2
Z
+∞
−∞
Z
S
2
Z
S
2
QT KAk
l
dθ
′′
dθ
′
dk
.
Similarly,
|W
n
| ≤ C sup
θ∈S
2
Z
+∞
−∞
Z
S
2
Z
S
2
QT KAk
l
dθ
′′
dθ
′
dk
Z
S
2
T KAdθ
′′
n
.
Finally,
Z
+∞
−∞
Z
S
2
Z
S
2
A(k, θ
′
, θ)dkdθ
′
dθ
≤
Z
+∞
−∞
Z
S
2
Z
S
2
˜q(k(θ − θ
′
))dkdθdθ
′
+C
0
kqk
2
L
2
(R
3
)
Z
S
2
T KAdθ
′′
+ 1
,
Z
+∞
−∞
Z
S
2
Z
S
2
A(k, θ
′
, θ)k
2
dkdθ
′
≤ sup
x∈R
3
|q|+C
0
kqk
2
L
2
(R
3
)
Z
S
2
T KAdθ
′′
+ 1
.
This completes the proof.
Lemma 11. Let
sup
k
Z
S
2
∞
Z
−∞
pA(p, θ
′
, θ)
4π(p − k + i0)
dp
dθ < α < 1/2, sup
k
pA(p, θ
′
, θ)
< α < 1/2.
Then,
|T
−
DΨ
0
| <
α
1 − α
, |T
+
DΨ
0
| <
α
1 − α
, |DΨ
0
| <
α
1 − α
,
T
−
g
−
= (I − T
−
D)
−1
T
−
DΨ
0
, Ψ
−
= (I − T
−
D)
−1
T
−
DΨ
0
+ Ψ
0
,
and q satisfies the following inequalities:
sup
x∈R
3
|q(x)| ≤
Z
S
2
T KQdθ
C
0
kqk
2
L
2
(R
3
)
+ 1
+ C
0
kqk
L
2
(R
3
)
.
Proof. Using the equation
Ψ
+
(k, θ, x) − Ψ
−
(k, θ, x) = −
k
4π
Z
S
2
A(k, θ
′
, θ)Ψ
−
(k, θ
′
, x)dθ
′
, k ∈ R,
we can write
T
+
g
+
− T
−
g
−
= D(T
−
g
−
+ Ψ
0
).
11

Applying the operator T
−
to the last equation, we have
T
−
g
−
= T
−
D(T
−
g
−
+ Ψ
0
),
(I − T
−
D)T
−
g
−
= T
−
DΨ
0
, T
−
g
−
=
X
n≥0
(−T
−
D)
n
Ψ
0
.
Estimating the terms of the series, we obtain using Lemma 4
|(T
−
D)
n
Ψ
0
| ≤
X
n≥0
Z
∞
−∞
. . .
Z
∞
−∞
Ψ
0
Y
0≤j<n
R
S
2
k
j
A(k
j
, θ
′
k
j
, θ
k
j
)dθ
′
k
j
4π(k
j+1
) − k
j
+ i0)
dk
1
. . . d
k
n
≤
X
n>0
2
n
α
n
=
2α
1 − 2α
.
Denoting
Λ =
∂
∂k
, r =
q
x
2
1
+ x
2
2
+ x
2
3
,
we have
Λ
Z
S
2
Ψ
0
dθ = Λ
sin(kr)
ikr
=
cos(kr)
ik
−
sin(kr)
ik
2
r
,
Λ
Z
S
2
H
0
Ψ
0
dθ = Λk
2
sin(kr)
ikr
= k
cos(kr)
i
+
sin(kr)
ik
2
r
,
Λ
Z
S
2
Ψdθ
=
Λ
Z
S
2
Ψ
0
dθ + Λ
Z
S
2
X
n≥0
(−T
−
D)
n
Ψ
0
dθ
>
1
k
−
α
1 − α
, as kr = π,
and
Λ
1
k − t
= −
1
(k − t)
2
Equation (2) yields
q =
Λ
H
0
R
S
2
Ψdθ + k
2
R
S
2
Ψdθ
Λ
R
S
2
Ψdθ
=
2k
R
S
2
T
−
g
−
dθ + k
2
R
S
2
ΛT
−
g
−
dθ + H
0
Λ
R
S
2
T
−
g
−
dθ
Λ
R
S
2
Ψdθ
=
2k
R
S
2
T
−
g
−
dθ + Λ
R
S
2
P
n≥1
(−T
−
D)
n
(K
2
− k
2
)Ψ
0
dθ
Λ
R
S
2
Ψdθ
=
W
0
+
P
n≥1
R
S
2
W
n
Λ
R
S
2
Ψdθ
.
12

Denoting
Z(k, s) = s + 2k +
2k
2
k − s
,
we then have
|W
1
| ≤
V p
Z
+∞
−∞
Z
S
2
Z
S
2
A(s, θ, θ
′
)s
s
2
− k
2
(k − s)
2
Ψ
0
sin(θ)dsdθ
k=k
0
≤
Z
+∞
−∞
Z
S
2
Z
S
2
Z(k, )˜q(k(θ − θ
′
))Ψ
0
dkdθ
+ C
0
Z
S
2
T KQdθ
.
For calculating W
n
, as n ≥ 1, take the simple trans formation
s
3
n
s
n
− s
n−1
=
s
3
n
− s
2
n
s
n−1
s
n
− s
n−1
+
s
2
n
s
n−1
s
n
− s
n−1
= s
2
n
+
s
2
n
s
n−1
s
n
− s
n−1
= s
2
n
+
s
2
n
s
n−1
− s
n
s
2
n−1
s
n
− s
n−1
+
s
n
s
2
n−1
s
n
− s
n−1
= s
2
n
+ s
n
s
n−1
+
s
n
s
2
n−1
s
n
− s
n−1
, (6)
As
3
n
s
n
− s
n−1
= As
2
n
+ As
n
s
n−1
+
As
n
s
2
n−1
s
n
− s
n−1
= V
1
+ V
2
+ V
3
.
Using Lemma 10 for estimating V
1
and V
2
and, for V
3
, taking again the simple
transformation for s
3
n−1
, which will app e ar in the integration over s
n−1
, we
finally get
|q(x)|
r=r
0
=
Λ
H
0
R
S
2
Ψdθ + k
2
R
S
2
Ψdθ
Λ
R
S
2
Ψdθ
k=k
0
,r=
π
k
0
≤
R
+∞
−∞
R
S
2
R
S
2
Z(k, )˜q(k(θ − θ
′
))Ψ
0
dkdθdθ
′
+ C
0
R
S
2
T KQdθ
(
1
k
0
−
α
(1−α)
)
+
Finally, we get
|q(x)|
r=r
0
≤ sup
x∈R
3
|q(x)|α + C
0
kqk
2
L
2
(R
3
)
+ C
0
kqk
L
2
(R
3
)
+
Z
S
2
T KQdθ
.
The invariance o f the Schr¨odinger equatio ns with respect to transla tions and
the arbitrar ine ss of r
0
yield
sup
x∈R
3
|q(x)| ≤
Z
S
2
T KQdθ
C
0
kqk
2
L
2
(R
3
)
+ 1
+ C
0
kqk
L
2
(R
3
)
.
13
4. Discussion of the three -dimensional inverse scattering problem
This study has shown, once again, the outstanding properties of the scatter-
ing operator, which, in combination with the analytical properties of the wave
function, allows us to obtain almost-explicit formulas for the potential from the
scattering amplitude. Furthermor e , this appro. The estimations following from
this overcome the problem of overdetermination, resulting fr om the fact that the
potential is a function of three variables, whereas the amplitude is a function
of five variables. We have shown that it is sufficient to average the scattering
amplitude to eliminate the two extra variables.
5. Studying the properties of solutions o f the Cauchy problem for the
Navier–Stokes equations using analytic functions generated by the
Sch r¨odinger equations and related to the Poincar´e-—Riemann—
Hilbert problem
Numerous studies of the Navier–Stokes equations have been devoted to the
problem of the smoothness of its solutions. A good ove rview of these studies
is given in Refs. [13–17]. The spatial differentiability of the solutions is an
important factor, as it controls their evolution. Obviously, differentiable so -
lutions do not provide an effective description of turbulence. Nevertheless, the
global solvability and differentiability of the solutions have not been proven, and
therefore the problem of describing turbulence remains open. It is interesting to
study the proper ties of the Fourier transform of solutions of the Navier–Stokes
equations. Of particular interest is how they can be used in the description
of turbulence and whether they are differentiable. The differentiability of such
Fourier transforms appears to be related to the appearance or disappearance of
resonance, as this implies the absence of large energy flows fr om small to large
harmonics, w hich in turn precludes the appearance of turbulence. Therefore,
obtaining uniform global estimations of the Fourier transform of solutions of the
Navier–Stokes equa tions means that the principle modelling of c omplex flows
and related calculations will be based on the Fourier transform method. We are
14

continuing to research these issues in relation to a numerical weather prediction
model; this paper provides a theoretical justification for this approach.
Consider the Cauchy problem for the Navier–Stokes equations:
∂~v
∂t
− ν∆~v + (~v, ∇~v) = −∇p +
~
f(x, t), div ~v = 0, (7)
~v|
t=0
= ~v
0
(x) (8)
in the domain Q
T
= R
3
× (0, T ), where
div ~v
0
= 0. (9)
The problem defined by (7)–(9) has at leas t one weak s olution (~v, p) in the
so-called Leray–Hopf class [16]. Denote:
µ(x) =
p
1 + |x|, |x| =
v
u
u
t
3
X
1
x
2
i
The following results have been proved [15]:
Theorem 4. If
µ~v
0
∈ W
1
2
(R
3
), µ
~
f(x, t) ∈ L
2
(Q
T
),
there is a single generalised solution of (7)–(9) in the domain Q
T
1
, T
1
∈ [0, T ],
satisfying the following conditions:
µ~v, µ∇
2
~v, µ∇p ∈ L
2
(Q
T
).
Note that T
1
depe nds on ~v
0
and
~
f(x, t).
Lemma 12 . Let µ ~v
0
∈ W
2
2
(R
3
), µ
~
f ∈ L
2
(Q
T
), then the solution of (7)–(9)
satisfies the following inequalities:
sup
0≤t≤T
||µ~v||
2
L
2
(R
3
)
+ ν
t
Z
0
||µ∇~v||
2
L
2
(R
3
)
dτ ≤ ||µ~v
0
||
2
L
2
(R
3
)
+ ||µ
~
f||
L
2
(Q
T
)
,
sup
0≤t≤T
||µ
~
∇v||
2
L
2
(R
3
)
+ ν
t
Z
0
||µH
0
~v||
2
L
2
(R
3
)
dτ
15

≤ ||µ ∇~v
0
||
2
L
2
(R
3
)
+ ||µ
~
f||
L
2
(Q
T
)
+
Z
t
0
||µ(~v, ∇~v)||
L
2
(R
3
)
||µH
0
~v||
L
2
(R
3
)
,
ν
t
Z
0
||µH
0
~v||
2
L
2
(R
3
)
dτ ≤ C +
1
ν
Z
t
0
||(µ~v, ∇~v)||
2
L
2
(R
3
)
dt.
Lemma 13. Let ~v
0
∈ W
2
2
(R
3
),
~
˜v
0
∈ W
2
2
(R
3
), and
~
f ∈ L
2
(Q
T
). Then, the
solution of (7)–(9) satisfies the following:
e
~v =
e
~v
0
+
t
Z
0
e
−νk
2
|(t−τ )
(
g
[(~v, ∇)~v] +
e
~
F )dτ,
where
~
F = −∇p +
~
f.
Proof. This follows from the definition of the Fourier transfor m and the theor y
of linear differential equations.
Let us introduce the op erators F
k
and F
kk′
as
F
k
f =
Z
R
3
e
i(k,x)
f(x)dx, F
kk′
f =
Z
R
3
e
i(k,x)−i(x,k
′
)
f(x)dx,
~
˜v(k) = F
k
~v,
~
V (k, k
′
) = F
kk′
~v =
Z
R
3
e
i(k,x)−i(x,k
′
)
~vdx.
Lemma 14. Let ~v
0
∈ W
2
2
(R
3
),
~
f ∈ L
2
(Q
T
), and |T KV
0
|+|T KV
0
|+
T K
2
V
0
~
˜v
0
<
C. Then, the solution of (7)–(9) in Theorem 4 satisfies the following inequali-
ties:
|˜v (k)| < C ,
|T K ˜v(k)| < C
0
||v||
L
2
(R
3
)
+
C
0
t
√
ν
||∇v||
L
2
(R
3
)
||v||
L
2
(R
3
)
.
Proof. This follows from
~
˙v = −(~v∇)~v + (ν~v + ∇p) + F,
~
˜v =
~
˜v
0
+
Z
t
0
e
−νk
2
(t−τ )
F
k
(− (~v, ∇)~v) + ∇p + F ) dτ.
From the last eq uation we have
|
~
˜v| = | ≤ |
~
˜v
0
| + C
T
.
16

Denote
β =
p
ν(t − τ), a = θx
formula 121 (23) from [11] as n = 0: yield
|T K~v| <
ke
−β
2
k
2
+
√
πβ
−1
e
−
a
2
8β
2
D
0
a
√
2β
,
|T K~v| ≤ |T K~v
0
|
+
T K
Z
t
0
e
−νk
2
(t−τ )
F
k
(−(~v, ∇)~v] + ∇p + F ) dk
≤ |T K~v
0
| +
Z
t
0
ke
−β
2
k
2
+
√
πβ
−1
e
−
a
2
8β
2
D
0
(
a
√
2β
)
||∇~v||
L
2
(R
3
)
dt
≤ C
0
||v||
L
2
(R
3
)
+
C
0
t
√
ν
||∇v||
L
2
(R
3
)
||v||
L
2
(R
3
)
.
Lemma 15. Let A > 0, p > 0, q > 0 1/p + 1/q = 1 then
V p
∞
Z
−∞
e
−Ak
2
k − l
dk
<
C
p
A
1/2p
+ C
0
e
−Al
2
V p
∞
Z
−∞
ke
−Ak
2
k − l
dk
<
C
p
A
1/p
+ C
0
(|l| + 1)e
−Al
2
Proof.
V p
∞
Z
−∞
e
−Ak
2
k − l
dk
<
V p
Z
|k−l|>|1
e
−Ak
2
k − l
dk
+
V p
Z
|k−l|<1
e
−Ak
2
k − l
dk
<
Z
|k−l|>1
e
−pAk
2
dk
1/p
Z
|k−l|>1
1
|k − l|
q
dk
1/q
+
V p
Z
|k−l|<1
e
−Ak
2
k − l
dk
V p
Z
|k−l|<1
e
−Ak
2
k − l
dk
<
Z
k=l+e
iφ
e
−Ak
2
k − l
dk
+ C
0
e
−Al
2
17

Denote
β =
p
(1 − cos(θ))(t − τ)ν
R = −F
kk′
(~v, ∇)~v] + F
kk′
∇p + F
kk′
F
Lemma 16. Let ~v
0
∈ W
2
2
(R
3
),
~
f ∈ L
2
(Q
T
), |T KV
0
| +
T K
2
V
0
~
˜v
0
. Then, the
solution of (7)–(9) in Theorem 4 satisfies the following inequalities:
|
~
V (k, k
′
)| < C, k|
~
V (k, k
′
)| <
C
p
(1 − cos(θ))
,
|T
~
V K| < C
0
||v||
L
2
(R
3
)
+
Z
t
0
1
β
Z
R
3
|x||v∇v|dx + ||∇v||
L
2
(R
3
)
||v||
L
2
(R
3
)
dτ
|T
~
V K| < C
0
||v||
L
2
(R
3
)
+
t
p
(1 − cos(θ))
max
0≤τ <t
Z
R
3
|x||v∇v|dx + ||∇v||
L
2
(R
3
)
||v||
L
2
(R
3
)
Proof. This follows from
~
˙
V = −F
kk′
[(~v, ∇)~v] + F
kk′
(ν∆~v + ∇p) + F
kk′
F.
After the transformations , we obtain
~
˙
V = −F
kk′
[(~v∇)~v] + (ν
k
F
kk′
~v + F
kk′
∇p) + F
kk′
F,
~
V =
~
V
0
+
Z
t
0
e
−νk
2
(1−cos(θ))(t−τ )
(−F
kk′
[(~v, ∇)~v] + F
kk′
∇p + F
kk′
F ) .
From the last eq uation, we have
|
~
V | ≤ |
~
V
0
| + C
0
Z
t
0
||∇v||
L
2
(R
3
)
||v||
L
2
(R
3
)
dτ.
Lemma (15) yield
T K
~
V
≤
T K
~
V
0
+
T K
Z
t
0
e
−νk
2
(1−cos(θ))(t−τ )
Rdτ
=
T K
~
V
0
+
Z
|k−l|>1
Z
t
0
e
−νk
2
(1−cos(θ))(t−τ )
|R|
k − l
kdkdτ
+
Z
|k−l|<1
Z
t
0
e
−νk
2
(1−cos(θ))(t−τ )
R
k − l
kdkdτ
18

I
0
+ I
1
+ I
2
;
I
1
<
Z
t
0
C
0
τ
(pν(1 − cos(θ))(1 − τ))
2/p
||∇v||
L
2
(R
3
)
||v||
L
2
(R
3
)
dτ.
I
2
=
Z
t
0
Z
|k−l|<1
e
−β
2
k
2
(R(k) − R(l) + R(l))
k − l
kdkdτ =
Z
t
0
Z
|k−l|<1
e
−β
2
k
2
R
′
(γ)k dk+R(l)
Z
t
0
Z
|k−l|<1
e
−β
2
k
2
k − l
kdk ≤
Z
t
0
|R
′
|
β
+
|R(l)|
β
!
dτ ≤
Z
t
0
1
β
Z
R
3
|x||v∇v|dx + ||∇v||
L
2
(R
3
)
||v||
L
2
(R
3
)
dτ ≤
t
p
(1 − cos(θ))
max
0≤τ <t
Z
R
3
|x||v∇v|dx + ||∇v||
L
2
(R
3
)
||v||
L
2
(R
3
)
Theorem 5. Let µ ~v
0
∈ W
2
2
(R
3
), µ
~
f ∈ L
2
(Q
T
),
~
˜
f ∈ W
2,1
2
(Q
T
), |T KV
0
| +
T K
2
V
0
~
˜v
0
< C,
R
∞
0
|µ|H
0
~
f||
L
2
(R
3
)
dt < C. Then, the solution of (7)–(9) in
Theorem 4 satisfies the following inequalities:
sup
0≤τ <T
|µ |∇~v||
L
2
(R
3
)
+ ν
T
Z
0
Z
R
3
µ|H
0
~v|
2
dxdτ ≤ const.
sup
x∈R
3
||~v(x)|| < C,
Proof. Consider the Cauchy pr oblem for the Navier–Stokes equations:
∂~v
∂t
− ν∆~v + (~v, ∇~v) = −∇p +
~
f(x, t), div ~v = 0, (10)
~v|
t=0
= ~v
0
(x) (11)
in the domain Q
T
= R
3
× (0, T ), where
div ~v
0
= 0. (12)
We perform the following transformations:
~u
ǫ
= ǫ~v, p
ǫ
= pǫ, f
ǫ
= f ǫ
2
, ν
ǫ
= ǫν, s =
t
ǫ
.
19

Then,
∂ ~u
ǫ
∂s
− ν
ǫ
∆ ~u
ǫ
+ ( ~u
ǫ
, ∇~u
ǫ
) = −∇
ǫ
p
ǫ
+
~
f
ǫ
(x, t), div ~u
ǫ
= 0, (13)
~u
ǫ
|
t=0
= ~u
ǫ
0
(x) (14)
in the domain Q
T
= R
3
× (0, T
ǫ
), where
div ~u
ǫ
|
t=0
= 0. (15)
Let us return for convenience to the notation v
i
= u
ǫ
i
, using the equation for
each v
i
= u
ǫ
i
. This gives us
−∆
x
Ψ + v
i
Ψ = k
2
Ψ, k ∈ C.
Using Lemmas 12 -15, we get estimates for
A
i
,
~
V
i
, T A
i
, T
~
V
i
, kA
i
, k
~
V
i
, T KA
i
, T K
~
V
i
, T K ˜v
i
, T K
2
V ˜v
i
.
The last es tima tions yield the represe ntation
q =
Λ
H
0
R
S
2
Ψdθ + k
2
R
S
2
Ψdθ
Λ
R
S
2
Ψdθ
|
r=
π
k
0
,k=k
0
,
and Lemma 11 implies
|µ |∇~v||
2
L
2
(R
3
)
+ν
ǫ
s
Z
0
||µH
0
~v||
2
L
2
(R
3
)
dτ ≤ ||∇µ ~v
0
||
2
L
2
(R
3
)
+
Z
s
0
||(µ~v)||
L
2
(R
3
)
||||µH
0
~
f||
L
2
(R
3
)
dτ
+
C
0
ν
ǫ
Z
s
0
max
x∈R
3
|~v|
2
||(µ∇~v)||
2
L
2
(R
3
)
dτ.
||µ∇~v||
2
L
2
(R
3
)
+ν
ǫ
s
Z
0
||µH
0
~v||
2
L
2
(R
3
)
dτ ≤ ||µ∇~v
0
||
2
L
2
(R
3
)
+
Z
s
0
||(µ~v)||
L
2
(R
3
)
||||µH
0
~
f||
L
2
(R
3
)
dτ
+
sC
0
ν
ǫ
Z
s
0
C
1
ν
ǫ
||(µ∇~v)||
2
L
2
(R
3
)
||(~v)||
2
L
2
(R
3
)
+ ||µ~v||
2
L
2
(R
3
)
||(µ∇~v)||
2
L
2
(R
3
)
dτ.
Denote
α(s) =
sC
0
ν
ǫ
C
1
ν
ǫ
||(µ∇~v)||
2
L
2
(R
3
)
||(µ~v)||
2
L
2
(R
3
)
+ ||µ~v||
2
L
2
(R
3
)
,
Z
T
ǫ
0
α(s)ds ≤
Z
T
0
T C
0
ǫν
ǫ
C
1
ν
ǫ
||(µ∇~v)||
2
L
2
(R
3
)
||(µ~v)||
2
L
2
(R
3
)
+ ||µ~v||
2
L
2
(R
3
)
dt
20

≤
T C
0
C
1
ǫν
3
ǫ
sup
t
||(µ~v)||
2
L
2
(R
3
)
Z
T
0
ν
ǫ
||(µ∇~v)||
2
L
2
(R
3
)
||dt +
C
0
ν
ǫ
sup
t
||(µ~v)||
2
L
2
(R
3
)
≤
T C
0
ǫ
4
ǫν
3
ǫ
+
C
0
ǫ
2
ν
ǫ
≤
2C
0
T
ν
= C
2
the Gronwall–Bellman le mma yields
||µ∇~v||
2
L
2
(R
3
)
+ ν
ǫ
T
Z
0
Z
R
3
|µH
0
~v|
2
dxdτ ≤ ||∇~v
0
||
2
L
2
(R
3
)
e
C
2
+e
2C
0
Z
T
0
||(µ~v)||
L
2
(R
3
)
||||µH
0
~
f||
L
2
(R
3
)
dτ.
Theorem 5 ass erts the global solvability and uniqueness of the Cauchy prob-
lem for the Navier–Stokes equations.
6. Discussion
As noted in the introduction, the key method of investigating the Cauchy
problem for the Navier–Stokes equations is its reduction to the Poincar´e–Riemann–
Hilbert pr oblem. By studying the wave functions for the Schr
¨
dinger equation
of the genera ted velocity components, we obtain unique estimates for the maxi-
mum veloc ity. Uniform global estimations of the Fourier trans form of solutions
of the Navier–Stokes equations indicate that the principle mode lling of complex
flows a nd related c alculations can be based on the Fourier transform method.
In terms of the Fourier transform, under both smooth initial conditions and
right-hand sides , no exace rbations appear in the speed and pressure modes. A
loss of smoothness in terms of the Fourier transform can only be expected in the
case of singular initial conditions or of unlimited for ces in L
2
(Q
T
). T he theory
developed by us is supported by numerical calculations performed in Refs. [18–
20], where the dependence of the smoothness of the solution on the oscillations
of the system is clearly deduced.
21
References
[1] Terence Tao, “Finite time blowup for a n averaged three-dimensional Navier-
Stokes equation,” -arXiv:1402.029 0 [ma th.AP]
[2] L. D. Faddeev, “The inverse problem in the quantum theory of scattering.
II”, Itogi Nauki i Tekhniki. Ser. Sovrem. Probl. Mat., 3, VINITI, Moscow,
1974, 93–180
[3] CHARLES L. FEFFERMAN E xistence and Smoothness of the Navier-
Stokes Equation. The Millennium Prize Problems, 57–67, Clay Math. Inst.,
Cambridge, MA, 20 06.
[4] J.S.Russell
≪
Report on Waves
≫
: (Report of the fourteenth meeting of the
British Association for the Advancement of Science, York, September 1844
(London 1845), pp 311—390, Pla tes XLVII-LVII)
[5] J.S.Russell (1 838), Report of the committee on waves, Report of the 7th
Meeting o f British Association for the Advancement of Science, John Murray,
London, pp.417-496.
[6] Mark J. Ablowitz, Harvey Segur Solitons and the Inverse Scatter ing Trans-
form SIAM, 19 81- p. 43 5.
[7] N.J.Zabusky a nd M.D.Kruskal (1965), Interaction of solitons in a colli-
sionless plasma and the recurrence of initial states, Phys.Rev.Lett., 15 pp.
240—243.
[8] R.G Newton , New result on the inverse scattering problem in three dimen-
tions, Phys. rev. Lett. v43, 8,pp.541-542,1979
[9] R.G Newto n , Inverse scattering Three dimensions,Jour. Math. Phys. 21,
pp.1698-1715,1980
[10] Somersalo E. e t al. Inverse scattering problem for the Schrodinger’s equation
in three dimensions: connections between exact and approximate methods. –
1988.
22
[11] Tables of integral transforms. v.I McGraw-Hill Book Company, Inc.1954
[12] Poincar´e H., Lecons de mecanique celeste, t. 3, P., 1910.
[13] Leray, J. (1934). ”Sur le mouvement d’un liquide visqueux emplissant
l’espace”. Acta Mathematica 63: 193–248. doi:10.1 007/BF02547354.
[14] O.A. La dyzhenskaya, Mathematic problems of viscous incondensable liquid
dynamics. - M.: Science, 1970. - p. 288
[15] Solonnikov V.A. Estimates solving nonstationary linearized systems of
Navier-Stokes’ Equations. - Transactions Academy of Sciences USSR Vol.
70, 1964. - p. 213 – 317.
[16] On global weak s olutions to the Ca uchy problem for the Navier-Stokes
equations with lar ge L-3-initial data Seregin, G; Sverak, V; NONLINEAR
ANALYSIS-THEORY METHODS and APPLICATIONS volume 154 page
269-296 (May 2017) Estimates of solutions to the perturbed Stokes system
[17] V. Vialov, T. Shilkin Notes of the Scientific Seminars of POMI, 410 (20 13),
5–24
[18] F. Mebarek-Oudina R. Bess a¨ıh, Magnetohydrodynamic Stability of Nat-
ural Convection Flows in Cz ochralski Crystal Growth. World Journal of
Engineering , vol. 4 no.4 , pp. 15–22, 2007.
[19] F. Mebarek-Oudina and R. Bes sa¨ıh, Oscillatory Mixed Convection Flow in
a Cylindrical C ontainer with Rotating Disk Under Axial Magnetic Field and
Vario us Ele c tric Co nductivity Walls, I. Review of Physics, 4(1 ) 45-51, 2010.
.
[20] F. Mebarek-O udina, Numerical modeling of the hydrodynamic stability in
vertical annulus with heat source of different lengths, Engineering Science
and Technolgy, an International Journal, 20, 1324-1333
23
